Quotient Rule for Derivatives

Suppose we are working with a function h(x) that is a ratio of two functions f(x) and g(x).

How is the derivative of h(x) = f(x)/g(x) related to f(x), g(x), and their derivatives?

Quotient Rule

Let f and g be differentiable at x with g(x) ≠ 0. Then f/g is differentiable at x and

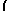
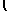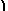
f(x) g(x)  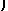′ = g(x)f ′(x)-f(x)g ′(x) [g(x)]2

Examples

• If f(x) = (2x+1)/( x-3), then

  f ′(x)	=	(x-3) d dx [2x+1] - (2x+1) d dx [x-3] [x-3]2
  	=	(x-3)(2)-(2x+1)(1) (x-3)2
  	=	- 7 (x-3)2 .

• If f(x) = tan(x) = sin(x)/cos(x), then

  f ′(x)	=	cos(x) d dx [sin(x)]-sin(x) d dx [cos(x)] [cos(x)]2
  	=	cos2 (x)+sin2 (x) cos2 (x)
  	=	1 cos2 (x)
  	=	sec2 (x),

  
  verifying the familiar differentiation formula for tan(x).

• If f(x) = 1/g(x), then

  f ′(x)	=	1 g(x)  ′
  	=	g(x) d dx [1]-(1)g ′(x) [g(x)]2
  	=	g(x)(0)-(1)g ′(x) [g(x)]2
  	=	- g ′(x) [g(x)]2 .

  For example,

d/dx[csc x]	=	d dx 1 sin(x)
	=	- d dx sin(x) [sin(x)]2
	=	-cos(x) [sin(x)]2
	=	-cot(x) sin(x)
	=	-cot(x)csc(x)

as expected.

Quotient Rule

Let f and g be differentiable at x with g(x) ≠ 0. Then f/g is differentiable at x and

f(x) g(x)

′

=

g(x)f ′(x)-f(x)g ′(x) [g(x)]2