in some classes at the beginning of basic school
… and, for example, to the use of squared paper →

How to differentiate the development of the various themes in different school levels. An example: the concept of angle
1. Premise
We want to ask ourselves the question of how to differentiate, for formalization and "technical" development, the teaching of the various themes in the different school levels. Let us bring out, a little "metaphorically", the various aspects of the problem referring to the development of a particular theme throughout the school period, or rather, the teaching of a specific concept.
2. The situation
Let's assume we're teaching maths in a strange school, where we will have to follow pupils from primary school to the end of secondary school. We have already made "setting" choices for the teaching of mathematics (they will appear in the following); right now we are delving into the reflection on how to distribute the development of the concept of angle over the years.
It is an unreal situation in many respects: we can design teaching without conditioning by "previous" or "later" teachers, only reasoning on a single mathematical topic (which in itself would make little sense, but, we repeat, it is a metaphor), ...; however, in its idealism it facilitates the highlighting of some important aspects of the problem of differentiation and the connection of the teaching of mathematics in different school levels.
We can, therefore, think "freely", referring to previous experiences, to our studies, ..., reading things that make us come up with ideas (even things of "educational research"), bearing in mind what the official school programs provide, ....
3. Angle and ...
Let's start by identifying some mathematical concepts that, sooner or later, we will have to weave to that of angle: direction, slope, measurement, rotation, half-line, polygon, circular functions, ....
We aim, in the reflection on the educational development of the concept, to take into account the uses of the angles (in various meanings) in other disciplines, in the experiences of daily life,....
We take into account our university and "personal" studies of mathematics (and physics and philosophy), from which we learned that geometry is an area with not well defined contours, which initially develops as a set of techniques (previously scattered, then organized) of direct and indirect spatial measurement, then intertwines with gnoseological and metaphysical reflections on the nature of space and time, ... and that its autonomy (in definitions and arguments) from physics is a relatively recent achievement, which passes through the numerical modeling of space by Fermat and Descartes, the gradual development of the concept of mathematical model and the focusing of the need to give an autonomous foundation to a science such as mathematics that has become of general use in industrial society, the delineation of the concept of formal system by Hilbert,….
Taking these aspects into account will help us to create effective educational itineraries with respect to the motivations and cultural needs of the pupils at the various school levels, to establish if/how/when to introduce definitions, demonstrations, activities on mathematical objects as such,….
4. Primary school (and kindergarten)
It is the first approach to school by pupils; It largely plays the possibility of initiating a deep cultural relationship (not only for the purposes of school evaluation or survival, on parallel or divergent tracks with respect to extracurricular experiences) with the pupil. We are therefore concerned to review the uses of the term and the concept of a angle (and related concepts) in daily life with which the pupil can have to do (corner of an object, "around the corner", "stay in a corner", ..., "turn right", "go straight", cardinal points, ... verbal communications, drawings, games, and various everyday activities); in English this ambiguity is partially overcome by using the words "corner" and "angle" differently; in other languages this is not possible.
It is a phase in which the child is enriching, with a high rate of growth, his linguistic-expressive baggage (relative to the natural language, to the "non-technical" drawing, ...). For this reason too, we must be careful not to make premature mathematical specializations of the meanings of the terms; the risk would be to foster conceptual confusions, burn expressive and cognitive potential, ....
We will also take into account that, at this stage of school, fortunately, we do not only teach mathematics, but also other disciplines: we can therefore prepare educational itineraries in which the mathematical aspect is naturally integrated with others and can be gradually brought out.
We work to find materials and reports of educational experiences that give us ideas and orientations to move in these directions (it is not easy: almost all the educational materials that can be found in a library are negative examples of what I would like to do).
5. Still primary school
As the first activities to which to refer the mathematical developments of the area of concepts connected with that of angle (see paragraph 3) we think, for example, of the description of paths followed by the pupils (to make "exits" from school, with some purpose – e.g. observe changes in a vegetable garden –, to go from home to school, ...). This context, in which you can integrate and/or do translations between spoken descriptions, descriptions with drawings, with photos, with first maps, ..., offers multiple opportunities to introduce, specify, delimit, ... the concepts that interest us: the directions of the stretchs of the path, , their slopes, the orientation of buildings, the ways in which the roads are grafted, the verses of the turns, the relativity of the references (compared to one that follows the path or with respect to the sheet or ...), the measure (what does a longer stretch mean? compared to time, as the crow flies, compared to the road to be traveled, ... or to the mood with which you have traveled it?), the shapes and proportions, ....
| Let's think back to some drawing activities carried out in some classes at the beginning of basic school … and, for example, to the use of squared paper → |
 |
Let's also think about other activities (easily inserted into various wide-ranging areas of work): the description of the movements of a person doing a certain activity, the description of how it is done and/or how a certain objectworks, … with various languages (verbal, iconic, mixed, …). Geometric transformations, new shapes, … also come into play.

The delimitation of meanings becomes important (for the pupils) in order, in these activities, to be able to communicate by understanding each other, to be able to pass from a verbal representation to a graphic one, …; reflection on concepts also helps to make things better; discover that sometimes there are ambiguities, that the same word can be used with different meanings, that in certain areas the words take on more restricted or different meanings from those used in common language, ... becomes a step towards organization (in sectors, disciplines , ...) of knowledge; ....
We think of resorting to activities in equally rich experiential areas to introduce the measurement of angles, use angles for more abstract modeling,, …: eg. the comparison between digital and analog time indication, the use of the clock and the compass to indicate the directions and to indicate the verses and the amplitudes (as differences of directions) of the rotations, the study of the phenomenon of shadows (which is the height of the sun? why and how does the shadow of an object deform as time passes or when changing the position of the object? ...), ...
|
|
|
6. What definition?
We've already got some ideas. Before better detailing the itineraries, we stop to reflect on how to eventually give the definition of "angle". We understand the importance of being gradual in the construction of the meanings of the various concepts and in the development of the lexicon, as well as in the "abstraction" of the performances required of the pupils (one thing is to face a situation directly, one thing is to face a situation real problem described, it is one thing to deal with an already formalized or pre-formalized problematic situation, such as the so-called "school problems").
Should we circumscribe the concept of angle by expressing a formal description in more abstract terms? Which?
How intersection of half-planes? But how can we define what a half-plane is? How could we naturally connect to the activities we intend to carry out (see paragraph 5)? And when did this definition of angle come about? in what context is it significant? ….
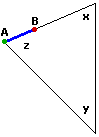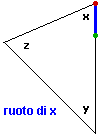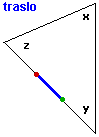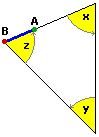
Then behind this concept is the idea of angle as "figure" in the sense of "part" of the plan, with all the risks of thinking (rightly, thinking of the common uses of "figure", of "part", ... and the ambiguous use of the word "side", now referring to segments, now to half-lines) at an angle as a kind of triangle (if it is a figure it will have a certain extension, finite, it is natural to think). We must strive to think about the meaning that children give to words and phrases, trying not to be influenced by our knowledge and our uses, other than those of the common language, which are the result of many years of study.

Could we define it as rotation (perhaps the introduction of the concept of rotation is easier than that of the concept of a half-plane)? We have read some proposals in this regard. But, rereading them, they leave us perplexed: it seems to us that we confuse the amplitudes of rotations, which are numbers, and angles, which are figures.
We are getting into issues that are not easy to get out of. We give up expetting to give a definition that exhausts the concept of angle. Moreover, we agree with what the programs suggest: in basic school geometry is to be understood as a gradual acquisition of orientation, recognition, localization, organization and schematization skills in/of the physical space. We will therefore limit ourselves to making students work appropriately in contexts that implicitly clarify the (abstract) meaning of the concept of angle (of Euclidean plane geometry). The contexts best suited to act as prototype situations seem to us those most corresponding to the idea of angle as part of a plane swept by a rotating half-line: if the half-line is a ray of light, the gaze or a rectilinear trajectory starting from a position fixed, ... it is possible to prevent the pupils from mentally representing the corners with limited parts of the plane. The situations on which we have thought to make the pupils work (see paragraph 5) seem suitable for this purpose.
Then, after having operationally introduced these concepts, we could also consolidate them also with animated images, easily made by the teacher, like these, or with dynamic geometry software, using ready-made files, which students can use for exploration and experimentation activities, such as those illustrated by the following images, relating to a file that allows to rotate AP or AQ around A and change its lengths (see here):

We could also use WolframAlpha (see):

A dynamic approach to the concept of angle allows us both to "conjecture" (see the figure below on the left) and the sum of the angles of a triangle is a flat angle, and to "prove it" (see the figure on the right).
 |  |
7. Lower secondary school
In the (strange) "6-18 years" school where we teach, at the beginning of secondary school some new pupils usually arrive, who have done primary school elsewhere. In planning the educational itinerary we must also take into account the problem of connecting our teaching to their knowledge and their attitude towards the school: how did they study? what do they know? what activities to explore this? what activities to insert them?
We will initially propose, to the whole class, some activities similar to those we have done in primary school. In this way we will try to address both an assessment of the productivity of our teaching and an analysis of the "level" of entry of new pupils, without "ad hoc tests", with which it would be difficult to explore their effective skills and would risk creating a fracture with other pupils. We will try, however, to insert moments of work to explore specific aspects, for example, still dwelling upon the concept of "angle", possible wrong conceptualizations of angles as limited figures (I could think of occasions when, faced with two angles of different width with the sides of the widest represented with segments shorter than those of the other - such as those of the first figure of paragraph 6 - the pupils must identify which is the widest angle).
8. What definition? - again
Part of the contents of the lower secondary school programs are already present in the basic school programs. Obviously, in the new school level we will have to start with a more formal and abstract presentation of the same. For the angle, developing what has already been observed in paragraph 6, we could use the concepts of half-line and rotation, which, in turn, we should somehow introduce.
How to introduce the half-line? The axiomatic path is certainly not feasible. We see no other way than to describe it through the idealization of a physical situation: the trajectory that (potentially) can be traveled starting from a certain position and without ever changing direction.
We will be able to give this description a greater formalization and/or make its abstract nature better perceived when we will introduce (relying on the experiences of reading and constructing graphs of phenomena already started in basic school) activities of graphical representation of functions and analytical representation of simple figures:
We will not speak of primitive notions (this would make sense only in the context of an axiomatic arrangement, in which the primitive terms and relations are those whose meaning is implicitly defined by the axioms, and is not left to intuition, as instead is found in many textbooks), nor will we attempt to give more formal definitions of "half-line" or "straight-line". Instead, we will take care, in the said way, to consolidate more abstract prototype situations in the pupils.
Moreover, if we try to understand, pretending not to know them already, the geometric (and algebraic) concepts using only the definitions present in various school books, we realize that we do not understand much from them: they are able to evoke ( to those who already know them) the concepts but not to individualizing them, being full of unspecified aspects, references to other concepts never defined, … . Perhaps the greatest difficulty of teaching is precisely that of decentralizing, of placing oneself from the point of view of those who don't already know things, but must acquire them through the activities and materials proposed by the teacher.
9. Still lower secondary school
Rotations, in the lower secondary school, are to be seen in the context of a more general presentation of geometric transformations. Also in this case it is important to keep in mind that we cannot ignore the references to physics: by working with geometric transformations the lexicon itself, the types of situations analyzed, … generally imply the presence of the time variable; while the steps of the translations are easily described in abstract terms (referring to the coordinates, without units of length), for the amplitude of the rotations we have to rely on physical measurements (with the protractor); ...
But we also teach science. And this "confusion" with physics is not a big disadvantage: on the one hand it allows us to make economies (you can deal with topics that belong to both disciplines in one fell swoop), on the other it allows us to clarify, by contrast, some characteristics of the various disciplines. For example, you can simultaneously deal with vectors to represent translations and vectors to represent displacements, and then focus on how vectors (with their addition) can be used as a model not only for subsequent movements (which can be confused with translations), but also for contemporary ones; this culturally very important step will allow us to start some reflections on the nature of mathematical models, on the use of geometric concepts in other areas, ...
We can also take other steps towards the mathematical clarification of the angle concept (and related concepts), in relation to the start of the first activities of analytical geometry. We have already mentioned this in paragraph 8. Another aspect is the quantification of the slope in the physical sense (ratio between the difference in height and the horizontal displacement) and the connection with the slope (angular coefficient) of the straight lines in the Cartesian plane (changing the horizontal scale or the vertical one changes the "physical" slope of the straight line y = 2 x, not the "mathematical" one). We begin o detach ourselves from the "protractor", and to introduce the "mathematical" concept of angle ..., and we begin to draw graphs and figures with the computer ...
| aspect ratio = 1 |  | aspect ratio ≠ 1 |
10. Upper secondary school
At the beginning of upper secondary school, link problems similar to those faced at the beginning of lower secondary school must be faced. But there is an additional problem. For the upper secondary school, without much variation, the same geometric contents already considered in the lower school programs are envisaged (a possible reflection on an axiomatic presentation can be addressed only at the end of the upper school), but it is not clear how it is possible to give an alternative presentation: geometric transformations are to be addressed only in an intuitive-synthetic way, as in middle school? must circular functions be restricted to convex angles? ... What to do?
In interpreting/translating the programs into didactic itineraries, it is necessary to see how to proceed in the transition from physical geometry to mathematical geometry. Meanwhile, as an alternative to the axiomatic presentation, we can refer to an analytical presentation of some basic concepts (point, movement, …) and then use them (in the development of geometry, in the proofs of some theorems, ...) by combining analytical and synthetic methods: respect in middle school the Cartesian plane will no longer be only a context to represent functions or to give algebraic shape to geometric concepts, but will become the (mathematical model of the intuitive concept of) "plane"; variables and equations will become not only tools for modeling relationships between real quantities but tools for defining new mathematical objects (geometric figures); the Pythagorean relationship will become the cornerstone for an abstract definition of distance; …
This change of perspective seems to us to be a significant differentiation from the lower secondary school. To achieve it, that is, to give a mathematical presentation to the concepts of movement, semirect, angle, ..., we will have to somehow give numerical form to the directions, and we will not be able to do without an introduction (not rigorous, but already "mathematical") to the concept of arc length (through a transition to the limit, a concept on which I will have already worked with the students dealing with the arguments of approximations and real numbers) and to circular functions in the full sense (sine and cosine as components of the versor, tangent as relationship between inclination and slope). This, moreover (wanting to be "good teachers") is inevitable, since first "physical" area activities have been planned.
 |
| The calculation of the arc AP length |
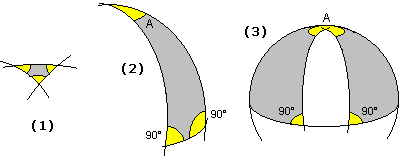
So that, at the end of upper secondary school, a reflection on the axiomatic approach becomes possible (but not only for this), as of now we will try, with respect to middle school, to start other abstractions. For example, we can highlight that, in mathematics, even "poorer" spaces can be used, where there is no mention of angles, where the points are in finite quantities, … (graphs to represent railway networks [see], road networks, ...); that a square represented in a non-monometric system may appear with different angles for the protractor; that different distances can be defined (with the urban distance the circle of center (0,0) and radius 1 appears graphically as a square); that the concept of equality is relative (to say that two triangles, or two other figures, that is, two sets of points, are equal as figures does not mean that they are equal as sets, otherwise they would be the same object, but that one can be transformed in the other with a movement or with an isometry or with a similarity or ... depending on the "geometric" considerations I want to make); that on a "spherical" terrestrial surface a pole and two points on the equator with a different longitude of 90° can be joined with three rectilinear paths forming a triangle with angles of sum 270°; …


 |
 |
| √(x²+y²) |x|+|y| | √(x²+y²) = 10 |x|+|y| = 10 |
 |  |
| ||
In this passage from intuitive geometry to formalized geometry, alongside the need to specify in an unambiguous way what the definitions are, the focus is to specify when an argument can be verified without a shadow of a doubt. In other words, the role and meaning of the demonstrations needs to be clarified. After doing it in simpler contexts, such as those of artimetics and the solution of the most basic equations (which are those that are addressed in mathematical logic courses), whose it is easier to focus and objectively verify the various steps with which it can deduce a property starting from others assumed as true or already demonstrated, in the case of geometry it is necessary to highlight the difficulty in carrying out arguments by separating the "suggested intuitions" from a figure (with which the most popular textbooks are full) from "controllable reasoning". We must be clear that the goal is not to demonstrate everything that is done, but to clarify the role of (definitions and) demonstrations.
11. In conclusion ...
Let's not continue this fantastic "simulation". Let us only mention, through some images, other "mathematical" (but not only "mathematical") contexts in which, in the didactic path, the concept of angle comes into play decisively:
 |
    |
|
|
|
|
Although we have touched only a few aspects, we hope to have underlined how, dealing, even concretely, in a specific didactic activity, relations with another school level, it is important to take into account how much and how the nature and role of mathematics must be outlined and specified compared to other disciplines and pupil knowledge systems. What is discussed here for the concept of "corner" can provide ideas for tackling other issues.